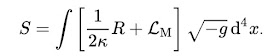Quantum mechanics of a black hole or a frozen star

Classically, the interaction between the mass elements dm of a frozen star slows down everything by an immense factor, and the factor keeps increasing as time passes.

Are there quantum mechanical aspects to this?

In our previous blog post we remarked that a black hole, or a frozen star, is not "static", because matter keeps falling closer to the event horizon, though the coordinate speed of the fall is minuscule. We argued that the Doppler effect causes the radiation out from a black hole to be much less than what Hawking radiation predicts.

The distance of a classical particle to the horizon may quickly become less than, say, 10⁻⁷² m in the Schwarzschild coordinates. In quantum mechanics, such short distances are nonsensical. How does quantum mechanics handle this?

The proper distance to the horizon is larger, though.

Dropping a small photon to the event horizon of a Schwarzschild black hole

Let us drop a photon of a wavelength 1 km to a black hole of 1 solar mass. The mass-energy of such a photon is

        h c / λ  *  1 / c²  =  2 * 10⁻⁴⁵ kg,

which corresponds to a Schwarzschild radius of

        2 G M / c²  =  10⁻⁷² m.

In quantum mechanics, the wave function of the photon is quickly squeezed into a volume which is 10⁻⁷² meters thick in the Schwarzschild coordinates and about 2 km wide.

What is the proper thickness of the volume?

The radial metric is stretched by a factor

       1 / sqrt(1  -  r / rs)  =  10³⁸.

Therefore the proper thickness of the volume is

       ~  10⁻³⁴ m.

Because of the blueshift, the wavelength of the originally 1000 m photon is only

       ~  10⁻³⁵ m

down there.

We see that the wave function of the photon "fits" easily into the thin layer above the old horizon. That suggests that the photon does behave much like a classical particle whose horizontal location on the event horizon has a large uncertainty of 1 km.

However, the further development of the photon becomes obscure in a classical treatment, because the photon itself affects the location of the event horizon.

The coordinate speed of light c' at the position of the classical photon particle is ~ 10⁻⁶⁸ m/s. If we assume that the speed does not become greater than that, then the position of the photon is essentially unchanged for the next 10³⁰ years, as seen by a faraway observer.

If we assume that we can determine the quantum behavior of the system by summing probability amplitudes of different "paths" of the photon, like in the Feynman diagrams, then the quantum behavior of the photon is much like the classical behavior.

When would the Hawking radiation formula hold?

     ------------------------------------------

    |                       |                       |

    |       M  T  λ           M  T  λ     |

    |                       |                       |

     ------------------------------------------

            "space"       "black hole"

Let us have two immense vessels filled with black body radiation, each vessel containing a mass-energy M. The temperature T of the black body radiation is the Hawking temperature of the Schwarzschild black hole of the mass M.

We connect the vessels with a hole whose radius is a few times the Schwarzschild radius of M, or a little shorter than the main wavelength λ of the black body radiation.

The system is in a thermodynamic equilibrium. Some radiation seeps through the hole into both directions. We may imagine that the vessel on the left is the "space" and the vessel on the right is the "black hole", and they are in an equilibrium. This would be a model of Hawking radiation, as Jacob Bekenstein and Stephen Hawking might have envisioned it.

However, the model is wrong. The black hole on the right is just forming, and presumably, almost all photons move to the direction of the black hole center, away from the hole. There is no equilibrium.

Note that since the photons are predominantly moving to one direction, they are ordered and have less entropy than black body radiation which would come from every direction. Here we have another example where lower entropy implies less radiation out.

Hawking radiation would require that the radiation on the right side would quickly "thermalize" with the walls of the vessel, and start coming equally from every direction. This is not something that we expect about a black hole. The radiation falling in does not have time to collide at the center and form "thermalized" radiation.

The myth of the event horizon "capturing" one of a virtual pair of particles

https://physics.stackexchange.com/questions/160765/what-are-the-virtual-particles-generated-during-the-hawking-radiation

In folklore, some people have tried to explain Hawking radiation by a mechanism where a virtual pair of particles is born and the event horizon captures the one with a negative energy. The black hole swallows the negative energy particle. The particle with positive energy is "freed" and escapes as a real particle. This real particle is supposed to be Hawking radiation.

Let us analyze this hypothesis.

1.   In this blog we hold the view that virtual particles only exist as temporary states when real particles collide. The hypothesis breaks this principle because the virtual pair is born from space which only contains a macroscopic, static, gravity field.

2.   In particle colliders we do not see any negative energy particles being produced. There is no empirical evidence that such particles can exist as independent particles.

3.   Nature seems to have a "transaction" mechanism which ensures that energy and momentum is conserved in all interactions. In the hypothesis, if the positive energy particle carries a momentum p and an energy E, then the negative energy particle carries -p and -E, and moves to the same direction as the positive energy particle. Why would the black hole swallow just one of these particles? How do we satisfy conservation of momentum and energy?

What is a virtual particle close to the event horizon?

Let us imagine a particle collider placed just at the event horizon. In Feynman diagrams, there are lines which represent virtual particles. What happens to these lines?

The particles in the collision are falling freely. In freely falling coordinates, the collision should happen in much the same way as in zero gravity, because of an equivalence principle. However, a faraway observer sees the process to "freeze", first from the lower side, and then entirely. The collision lasts very long in coordinate time, maybe forever.

     

                     wave 1

          -----------~~~~----------  field 1

                         |   interaction

          -----------~~--------------  field 2

                     wave 2

What is a frozen virtual particle? In this blog our view is that a virtual particle is not a free particle, but an interacting particle. In the wave interpretation, two or more waves are interacting.

The waveform of a virtual particle is not the ordinary sine wave, but can be distorted in many ways. This explains why a virtual particle can carry an arbitrary amount of energy and momentum.

Thus, a frozen virtual particle is simply a snapshot of the collision. The field is twisted to a form which only last for a very short proper time in the eyes of a co-falling observer. A faraway observer may measure that to last forever.

Could it happen that a negative energy virtual particle is frozen at the horizon and a positive energy real particle escapes?

Negative energy particles in a Feynman diagram

https://en.wikipedia.org/wiki/Vacuum_polarization

Above we have a vacuum polarization loop in a Feynman diagram. Time progresses from left to right, the wavy line is a photon, and the loop is a virtual electron-positron pair which is created and annihilated.

The positron and the electron may have arbitrary energies in the loop, as long as the sum of their energies is the energy of the photon.

Conclusions

https://en.wikipedia.org/wiki/Schwinger_effect

Let us close this blog post. We will next look at Feynman diagrams, or other arguments, which might support the existence of the Schwinger effect or the existence of Hawking radiation. In both cases, the crucial question is if something like a "negative energy particle" can exist independently.

A negative energy particle is a formal calculation tool in a Feynman diagram. There is no empirical evidence that such a particle is a genuine natural phenomenon. It might just be a term in a calculation formula.

We have collected many arguments against the existence of Hawking radiation. The Schwinger effect brings a new aspect to this.

The Bekenstein bound of entropy

UPDATE June 25, 2024: Did we refute thermodynamics, too? If we have a solid block of matter at absolute zero, and warm it up with random photons of a very long wavelength, it will later radiate away the heat as photons of a higher energy. The number of photons is reduced.

Why is entropy conserved? Maybe because the time at which the infrared photons leave, adds more entropy?

Or is it so that a short wavelength photon carries away more information because of the short wavelength?

Maybe it is best to check if a perpetuum mobile can be constructed with Hawking radiation. A perpetuum mobile is well defined, while "information", or entropy, is not.

Let us have a large vessel full of photon gas at a low temperature. We squeeze the vessel until the photon gas collapses into a black hole. After that, the hypothetical Hawking radiation will make the black hole to evaporate. The final stage of the evaporation releases photon gas in a violent explosion. Does this allow us to construct a perpetuum mobile?

----

What is the maximum entropy that a mass M of matter can contain if the matter is in a volume V?

This is the question in the Bekenstein bound. 

If we feed a black hole with photons whose wavelength is ~ 17 X the Schwarzschild radius, then the black hole will probably contain the Bekenstein bound worth of entropy.

https://en.wikipedia.org/wiki/Bekenstein_bound

Maximizing the number of individual particles and minimizing the frequency f

The way to increase entropy is to divide the mass M to the maximum possible number of particles, typically photons. The wavelength of the photons is restricted by

        λ  <  2 ∛ V,

if V is a cube.

The energy of a single photon is

        h f,

where h is Planck's constant and f is the frequency. If we can make the speed of light c slower inside V, then each photon has less energy, even though its wavelength is the same as before.

We can attempt to increase the entropy inside V by slowing down light inside V. This is what gravity does.

Using phonons in a solid

Let us have two million low-energy particles. We build a solid material from one million particles. We assume a potential of a form k x² between those particles. The energy of the rest 1 million particles we insert into the solid as phonons.

The solid has to withstand an enormous energy which is put into phonons: the energy is as large as the mass-energy of the solid. We have to make k extremely large, which, in turn, means that the speed of sound is close to c.

Also, since the solid only has some 100³ particles, we cannot have short wavelengths in the phonons.

It does not look like that using a solid and phonons can increase the entropy, compared to a photon gas.

Feeding a black hole with extremely long wavelength photons: there is no Bekenstein bound

Is there a reason why a black hole would be unable to absorb photons whose wavelength is much larger than 17 times the Schwarzschild radius?

The probability of absorbing such a photon is very low, but not zero? What is the waveform like close to the event horizon of a Schwarzschild black hole?

https://en.wikipedia.org/wiki/Schwarzschild_metric

The metric is:

where

        rs  =  2 G M / c²,

if M is the mass-energy of the black hole measured from far away.

The local proper time close to the event horizon runs at the rate

       sqrt(1  -  rs / r)

relative to the coordinate time, or the time of a distant observer.

The proper radial length is stretched by the factor

       1 / sqrt(1  -  rs / r)

relative to the radial coordinate r length. Thus, the coordinate speed of light, c', is only 

        c'  =  (1  -  rs / r) c

close to the horizon.

If we send a wave of a length λ from a radial coordinate R toward the horizon, there will be

              R

       ~   ∫  1 / (1 - rs / r)  *  dr

          rs 

       =  ∞

cycles before the wave reaches the horizon.

If we have a very long wavelength far away in space, the wavelength will be tiny relative to the size of the black hole as the wave approaches the horizon. It is plausible that a photon of such a wavelength can be captured by the black hole.

We can feed the black hole with photons whose energy is arbitrarily small. This proves that the entropy can be arbitrarily large. There is no Bekenstein bound for black holes!

Feeding very small energy photons to a black hole through a harness around the black hole

Above we calculated that a very long wavelength photon has a reasonably short wavelength close to the horizon of a black hole.

Let us build a harness around the black hole such that it is relatively close to the horizon. Now we can efficiently feed photons of a very small energy into the black hole.

The information content, or entropy, is a vague concept

Above we proved that we can feed a black hole with photons of a very long wavelength. But is their "entropy" really very high?

We can heat up a block of solid material with very long wavelength radio waves. Later, the block will radiate away infrared photons which have a much shorter wavelength. There is no contradiction in this. The number of photons can decrease.

An attempt at constructing a perpetuum mobile which converts heat into mechanical energy

Let us have a vessel full of photon gas which is black body radiation for an extremely low temperature T. Let the gas be in a thermodynamic equilibrium with a black hole inside the vessel, assuming thst Hawking radiation exists.

A typical Hawking photon has a wavelength of 17X the Schwarzschild radius.

If we want to squeeze the photon into a "package" which can easily be dropped into the black hole, we have to make the wavelength 1/17 of the original. Thus, N might be 17X M. Can we harvest so much mechanical energy in a cycle?

As we lower the package closer to the event horizon, the photon is blueshifted. Does this allow us to squeeze the package smaller?

We could have an observer sitting close to the horizon. Now and then he will catch a very much blueshifted photon. He may even be able to make particles with a positive rest mass from the radiation hitting him from up and down.

The observer can then lower a particle with a positive rest mass closer to the horizon and harvest some mechanical energy.

Did we just prove that it is possible to harvest mechanical energy from a system which is in an equilibrium?

The same argument can be used for an ideal gas. Sometimes, a gas atom with a very high kinetic energy will hit an observer, and he can harvest some mechanical energy. But that is not considered a perpetuum mobile.

The hypothetical Hawking radiation causes radiation pressure. If we have a container of a volume V close to the horizon, the pressure from downward is larger than from upward. The gravity of the radiation inside the container probably makes up for the difference. It is like an atmosphere in an equilibrium. We cannot harvest energy by moving a container full of gas up or down.

Any gravitating body whose temperature is above absolute zero and which is inside a vessel in a thermodynamic equilibrium, possesses a similar atmosphere of infrared photons. This is not unique for a black hole.

The Hawking temperature

https://en.wikipedia.org/wiki/Hawking_radiation

The temperature for a solar mass black hole is 60 nanokelvins. Wien's displacement law gives 50 km or 17 rs as the typical wavelength of the infrared radiation.

The Hawking formula says that the black hole is in a thermodynamic equilibrium with black body radiation whose typical wavelength can just slip through the "hole" in space.

Is this reasonable?

Let us imagine an extremely strong rigid shell which encloses the black hole, very close to the horizon. What is the equilibrium temperature of the shell?

We probably get the temperature from the blueshift and Wien's displacement law. The power of black body radiation is ~ T⁴. One factor of T is explained by the blueshift, the rest T³ is probably explained by the fact that the strong gravity field pulls most photons back to the surface of the shell. Only photons leaving approximately vertically can escape.

A collapse of a photon cloud

Let us make a black hole by letting dust to collapse. The lightest possible speck of dust is a photon. The "kinetic energy", or heat energy, of the a photon is its own energy. Under these assumptions, the lowest temperature that the cloud of dust of a certain size can have is roughly the Hawking temperature T.

If we let the photon cloud collapse, does the temperature for an observer far away appear the constant T regardless of the phase of the collapse? The Hawking radiation hypothesis would suggest this.

Let the surface of the cloud fall to a potential

       U(r) << 1,

where f / U(r) is the frequency of a photon of a frequency f sent by a static observer at the radius r.

If the temperature T(r) of the surface goes as

       ~  1 / U(r),

and the surface of cloud is static as it falls (it cannot be), then a faraway observer would see the temperature as the constant T.

But the surface of the photon cloud is falling down, eventually at a velocity which is almost the local coordinate velocity of light.

The Doppler effect will make the wavelength of the outgoing radiation much larger than in the case of a static cloud surface. A long wavelength wave has problems escaping from the small "hole" around the forming event horizon. Furthermore, the energy of escaping photons is reduced by the Doppler effect.

We conclude that the radiation that a faraway observer sees will have an intensity much less than the black body radiation of the temperature T. The main wavelength of the outgoing radiation will be much larger than 17 rs.

Here we have yet another strong argument against the existence of Hawking radiation. A collapsing photon cloud will radiate much less than what the Hawking radiation would be.

If the Hawking radiation exists, when will it start? Our outline of the process does not indicate any moment when Hawking radiation should start.

Conclusions

It is hard to define what is the information content, or entropy, for a given flux of radiation. One could claim that a single photon can code an infinite amount of information.

We then looked at a better defined problem: can one use Hawking radiation to construct a perpetuum mobile? It looks like a perpetuum mobile is not possible. Hawking radiation forms an "atmosphere" around a black hole, and one cannot make a perpetuum mobile from the atmosphere of a celestial body.

We then turned to studying a collapse of a photon cloud. We could as well study a collapse of particles with a non-zero rest mass. The surface of the collapsing clouds falls almost at the speed of light. The Doppler effect reduces the outgoing radiation intensity dramatically. This does not agree with the Hawking hypothesis at all. There should be a flux of black body radiation at a fixed temperature T.

In principle we could stop the collapse process if we had superstrong materials. If Hawking radiation would exist, we would have hard time explaining from where did it get its energy.

We obtained new strong arguments against the existence of Hawking radiation.

If the black hole would be a static system in a thermodynamic equilibrium with the surrounding space, then it looks likely that it would radiate at the temperature T. But a black hole, or a frozen star is not static, at least if we look at it as a classical object. Does quantum mechanics bring another aspect to this?

Disassembling a black hole

Our previous blog post about a frozen star opens a way to disassemble a frozen star – or a black hole – into its components. Our solution is to accelerate the frozen star M linearly so violently that the particles that are falling into at the back of M will feel a "force" away from M.

                   m falling in

                    • -->    ● ---->  extreme acceleration

                              M frozen star

In the Minkowski & newtonian model, the gravity force, measured in the canonical coordinates of Minkowski space, is not infinite close to the event horizon. The force is only "reasonably" strong, like that of the newtonian gravity force of M, or some small multiple of that.

The inertia of the test mass m resists the acceleration of m along with M. How big is the inertia of m in this case?

Let us pull the system M & m with a large force F. What is the inertia of m in this operation? We assume that m fell from a large distance close to the event horizon of M. A reasonable guess is that the inertia of m is simply m.

Thus, if we accelerate M fast enough, then m in the accelerating frame of M will feel a force to the left. The test mass m has a very large inertia when it is close to the horizon. The test mass m will slowly climb up, so tht its distance from M grows. Essentially, this is the reverse of the descent of m toward the horizon of M.

We showed that we are able to lift a falling mass m up from the horizon of M. We can then iterate this process and disassemble M by pulling eventually all particles up from M.

Though, in practice, we are unable to perform the disassembling. It would require a huge acceleration of a black hole.

Hawking radiation cannot exist

Through disassembling we can, in principle, retrieve all the information which fell into a black hole, or a frozen star. This is strong evidence against the existence of Hawking radiation. A black hole does not destroy information. The information is, in principle, retrievable. It would be very strange if Hawking radiation could mysteriously destroy this information.

The resolution of the famous black hole information paradox is that Hawking radiation does not exist.

In this blog we have harshly criticized the hypothesis that Hawking radiation exists. Stephen Hawking used a wrong interpretation of creation operators in (scalar) quantum field theory.

Hawking radiation seems to break unitarity, which is very strong evidence against its existence.

Also, it is not clear how Hawking radiation could obey conservation of energy and momentum.

The temperature of a black hole

A frozen star contains a lot of information, or entropy. Should the star radiate infrared because it has this entropy?

Let us consider a block of glass. Since it is amorphous matter, it contains a lot of entropy or information. Let us cool the block of glass very close to absolute zero. Does the block of glass radiate infrared?

The atoms in the glass will extremely slowly arrange themselves into crystals, releasing some heat. This heat will radiate away. But the flux of infrared is extremely slow.

Is there any lower limit how much infrared should some amount of entropy S radiate?

The Bekenstein hypothesis of entropy: the Bekenstein bound

https://en.wikipedia.org/wiki/Black_hole_thermodynamics

The hypothesis of Jacob Bekenstein claims that a black hole has an entropy which is proportional to the area of the horizon. 

The hypothesis is suspicious. If we throw a crystal into a black hole, why should the entropy of the falling crystal grow to match the (large) Bekenstein value for a black hole?

Also, is there any proof that no ordinary object can have an entropy greater than the Bekenstein value?

If we have one kilogram of noninteracting photons in a cubic meter, one can use quantum mechanics to calculate the number of possible microstates. The value probably agrees with the Bekenstein hypothesis.

http://www.phys.huji.ac.il/~bekenste/PRD23-287-1981.pdf

What happens if we add interactions? Intuitively, the system is now more "complex", and there might be many more microstates available. For example, in a solid, there are sound waves. Jacob Bekenstein in his 1981 paper ignores interactions.

If we add one very low-energy photon into a vessel whose size is a cubic meter, the photon can take only a few different states, because its wavelength is ~ 1 meter.

If we add a phonon of the same energy into a cubic meter of a solid, the wavelength is much less than that of the photon. It has a huge number of different states.

https://en.wikipedia.org/wiki/Bekenstein_bound

Wikipedia claims that the Bekenstein bound for the entropy of 1 kg in 1 m³ was proved by Horacio Casini in 2008, in quantum field theory:

https://arxiv.org/abs/0804.2182

Since the problem is very general, it is doubtful that one can solve it in quantum field theory alone.

The temperature T of a system with entropy under a low gravity potential: no infrared radiation is necessary

Let us have a block B of matter at some temperature T. It radiates a certain black body infrared spectrum at a power W.

We move the block B onto the surface of a very heavy neutron star. The spectrum of the block B is greatly redshifted, and the radiation power W' is now much less than W.

If we put B into ever lower gravity potential, is there a limit how close to zero can W' be?

Apparently, not.

There is no requirement that a black hole or a frozen star should radiate infrared at all. The local time there runs extremely slowly. Thermodynamics does not say that something like Hawking radiation should necessarily exist.

Conclusions

We sketched a method which can, in principle, be used to disassemble a black hole or a frozen star.

This resolves the famous black hole information paradox: the information is preserved inside the frozen star, and Hawking radiation does not exist.

We will do more research on the Bekenstein bound. Do interactions allow to store much more entropy into a 1 kg mass in a cubic meter?

Star collapses into a "frozen star"

Now that we understand the shortcomings of general relativity better, let us revisit the problem of what happens when a star collapses into a black hole.

Collapse of a spherical shell M of dust

     

       M

           --> v                                    <-- v

        • • • • •                ×                • • • • •

          |                                              |

          rs                                             rs  

         

Let us assume that the initial mass-energy of the shell is M, measured from far away. The shell is thin.

The outer edge of the shell M approaches the radius rs(M) where the redshift of a photon sent upward into space becomes infinite. The speed of light, as observed by a faraway observer, is zero at rs(M).

The radius rs(M) is the Schwarzschild radius of M in general relativity. However, in this blog post we do not assume that general relativity is true. The gravity model can be different.

Definition of the gravity "potential". The gravity "potential" of a test mass m at a radius r is

       V(r)  =  E / m,

where E is the energy which can be recovered if we convert m to photons and send them to faraway space. The potential is not defined if photons cannot be sent to faraway space.

The shell is thin. It is reasonable to assume that the outer edge approaches rs(M) earlier than some inner subset M' ⊆ M approaches its respective radius rs(M').

Approach of the outer edge to rs(M) takes an infinite time

Let

       V(r)

be the potential at r once the entire shell M has falled inside the radius r.

Assumption 1. The potential V(r) falls essentially linearly at rs(M).

Then the local speed of light c', as measured by a faraway observer, is:

       c'  ~  r  -  rs(M).

A faraway observer measures that the time to reach rs(M) is

           rs(M)

       ~  ∫      1  /  (r - rs(M))  dr

         r₀

       =  ∞.

Local versus global time inside M

The coordinates (t, r, φ, θ) that we use can be imagined as the view of a faraway observer.

Assumption 2. The local proper time inside the outer edge of the shell M runs at most at the speed of the empty space just out of the outer edge. The "speed" of the local time is defined relative to the global time coordinate t.

As long as one can send photons to faraway space, Assumption 2 is true. A photon sent from inside the shell M necessarily has a larger redshift than a photon sent from the outer surface of the shell M.

If photons cannot be sent, then we take the assumption as an axiom. One can imagine that something "happens" inside the shell M, even if a photon never can reach the space outside M. An analogous case is a box made of metal. One cannot send a photon out of the box, but there certainly happens something inside the box.

The local speed of light c' can approach zero so fast that a photon from an event X cannot ever reach faraway space. But we can still develop the system forward in time.

Also, we may be able to define the global time coordinate from local phenomena. If a photon can be sent to a close location y from a location x, and back from y to x, then we can synchronize the global time at x and y. If local time runs slower and slower inside M, we may still be able to synchronize global time at locations

       x₀, x₁, ... xn,

where x₀ is inside M, and xn in faraway space.

The fate of the collapsing shell: a frozen star

It takes the outer surface of the shell M an infinite time to reach rs(M). The speed of light c' inside M approaches zero extremely fast.

The interior of M is essentially frozen. No singularity can form.

A collision of two frozen stars: a collision of two chunks of "syrup"

In this blog we have earlier investigated the collision of two frozen stars. We had the "syrup" model of gravity, in which two large chunks of syrup can move fast, even though a small test mass m is frozen at its position inside the syrup. We wrote about the merger of two black holes on June 26, 2023.

The frozen star of the section above can be imagined as syrup whose viscosity is increasing extremely quickly.

But we can still accelerate the star, and make the entire star to move very fast. What happens if two such chunks M of syrup collide?

            __              __

          /    \          /    \

         |       |         |       |

          \__/           \__/

          --> v         v <--

           M              M

The speed of both chunks is relativistic at the collision. They have length contracted.

Let us call an event horizon a surface around both M, such that the redshift from the surface is extremely large.

Is it so that both chunks of syrup will sink quickly below, or extremely close their common event horizon?

                    |

                • • • • •   --> particles falling in

                • • • • •

                    |

              horizon

The event horizon cannot "support" anything a large coordinate distance above the horizon?

The radial spatial metric is very much stretched close to the horizon in general relativity.

In the diagram above, each particle • is falling at a relativistic local proper speed. The local speed of light in global coordinates t and r becomes extremely slow close to the horizon. The distance of each particle from the horizon probably falls exponentially in t.

Thus, all the particles will very quickly come extremely close to the horizon, which also moves outward as more particles fall in. Many particles end up below the horizon.

We conclude that both masses M very quickly end up either inside the horizon, or extremely close to the horizon.

What is the form of the horizon, after some global time t has passed?

An extremely slow speed of light c' close to the horizon distort greatly the lines of force of the (newtonian) gravity field. In many gravity models, the horizon will be perfectly spherical. This is because the distortion tends to pull oblique lines close to the horizon. Outgoing lines of force must go radially straight from the center of the system. The energy of the field in many models is then minimized by uniformly spaced lines of force leaving a perfectly spherical event horizon.

In particular, the event horizon is spherical in general relativity.

There may be event horizons embedded inside each other. The horizons of the individual masses M are, in some sense, preserved under the larger, common event horizon.

The field of a rotating merger of frozen stars

              ^

              |               M

              ●               ●

             M               |

                               v

Let us then analyze what happens in a collision where two frozen stars M initially orbit each other.

This is a much more complicated setup than a head-on collision. Some aspects:

1. How does gravitomagnetism or frame dragging affect the process?

2. What is the formula for gravitomagnetism?

3. What formula should be used for the centrifugal "force"?

4. Is the gravity potential newtonian, or is it steeper as in general relativity?

5. Once the stars have merged, is the gravity field rotationally symmetric, and does not radiate quadrupole waves any more?

The LIGO group used computer models to calculate approximate solutions. Our May 21, 2024 results show that the Einstein field equations do not have a solution for any "dynamic" system. Thus, the LIGO group is not solving the Einstein equations, but something else. They are, at least, calculating corrections to the newtonian orbit from the Schwarzschild metric around each orbiting mass.

Each frozen star M is very viscous "syrup", but as a whole, each star can move (and probably spin) like any other body floating in space. Therefore, the initial approach of the stars can be handled with post-newtonian approximations and the slowdown which comes from the loss of energy and momentum to gravitational waves.

As the stars come closer to each other, so that they would be partially inside their common event horizon, what happens then?

In a preceding section we argued that any matter outside an event horizon can approach the horizon extremely quickly, so that the distance shrinks exponentially, as measured by a faraway observer.

If that is the case, we still have to prove that the gravity field approaches very quickly a rotationally invariant form. Otherwise,we would not have the quick "ringdown" in the LIGO observations.

The gravity field of a nonrotating frozen star is spherically symmetric

In our blog we have earlier argued in the following way for a non-rotating black hole: Since the potential is very low close to the event horizon, or inside it, we can essentially draw the lines of force of the newtonian gravity field as we like inside that volume. Twisted lines inside add very little to the energy of the field, since they are at an almost zero potential (the redshift is extremely large).

Then, the way to minimize the energy of the newtonian field is to draw the lines of force at a uniform density from a spherical surface enclosing the mass. The energy of the field is dominated by the lines of force which are outside the event horizon.

The event horizon cannot be inflated arbitrarily far from the black hole because the gravity potential has to be zero at its surface.

This is similar to adding an electric charge to a flexible metal mesh. The smallest energy is attained when the mesh is inflated to a spherical shape, and the electric field lines emanate at a uniform density from the surface.

This analysis holds for many gravity models. The essential thing is that the field wants to find its minimum energy just like a Coulomb field, and the gravity potential is very low at the surface of the mass configuration. In general relativity, a very heavy, and an extremely rigid neutron star already has a gravity field which is spherically more symmetric than the star itself.

The rotating gravity field of two merged frozen stars

Let us assume that a common event horizon already encloses the two merged stars.

If the rotation is slow, then it seems plausible that the event horizon is almost spherical and the gravity field is almost spherically symmetric.

For a rapidly rotating merged frozen star, or a merged black hole, the energy of the gravitomagnetic field has to be added to the energy of the Coulomb-like newtonian gravity field. The newtonian gravity field lines should be less dense at the equator. 

What about the rotational symmetry?

The field energy outside the horizon is probably minimized by making the field symmetric around the rotation axis. The field lines "repulse" each other, and the horizon forms where the redshift approaches infinity.

                   ω

                   -->

                   ●●  merged frozen stars

              

The merged frozen stars may form a rotating dipole inside the common event horizon, like in the diagram above. But their gravity field will be rotationally symmetric outside the event horizon. Therefore, very soon after the merger, gravitational waves are no longer emitted.

Since the newtonian gravity field lines are sparser along the equator of the rotating system, the event horizon has a smaller radius at the equator than at the poles of the rotating frozen star.

The effect of the centrifugal "force"

The analysis is complicated by the centrifugal "force" at the equator of a rotating frozen star. How does it affect the event horizon?

If we shoot a rocket of a mass m out vertically from a launchpad on the equator of Earth, the rocket gets

       1/2 m * (460 m/s)²

of kinetic energy from the rotation of Earth. The escape velocity is reduced, thanks to the extra kinetic energy.

If we shoot a photon vertically from the launchpad, the Doppler effect blueshifts it. 

The event horizon has an infinite redshift. Can the Doppler effect affect the radius of the horizon at all?

Yes, it can. The horizon is at the radius where the potential of a test mass is zero. The centrifugal "force" can cancel a significant part of the gravity attraction.

Another aspect is the time dilation. If the source of a signal moves at a large velocity on the event horizon of a frozen star, then there is a significant "redshift" from the time dilation.

What is the local rate of time in an accelerating system? Where is the event horizon located for a rotating mass M?

Let us make the following thought experiment:

               v <-- •  ● ---->  rapid acceleration

                      m M

We have a test mass m close to a nonrotating frozen star M. Then we pull M extremely fast to the right. The escape velocity of m to the left is then surprisingly slow. Does this mean that the "potential" at m was surprisingly high, and the local time at m ran surprisingly fast.

Obviously, not.

In a dynamic system like above, it is hard to define the gravity "potential". The local rate of time cannot then be bound to the potential.

In the static Schwarzschild metric, the rate of local time is determined by the potential. Why? Is it a coincidence?

According to our Minkowski & newtonian model, the slow rate of a mechanical clock close to M is due to two factors:

1. the inertia of the clock parts is larger, and

2. the energy stored into a spring in the clock is less because the spring is at a low gravity potential.

Now we face a dilemma: how do we define item 2 in a dynamic system?

Maybe we should calculate the "current" potential of m in the field of M, and ignore any acceleration.

What if M is rotating? Frame dragging may force m to move along with the surface of M. Can we still ignore the acceleration of the mass elements in M?

For a rotating electric charge Q, the rotation creates a magnetic field B, but it does not affect the electric potential.

               • --------> •     rotating heavy mass M

              ^              |  

              |              v

              • <--------- • dm

                    <-- • m test mass

Let us analyze the diagram above. The rotating mass M is very heavy and the local coordinate speed of light c' is very slow. The test mass m must move along the surface of M.

What is the gravity "potential" at m? Let m carry a mechanical clock. Its parts have a lot of extra inertia. What is the energy available for the springs of the clock to move the clock parts?

The simple guess is that we have to add the negative potentials of each mass element dm in the large mass M, to obtain the potential at the test mass m.

In our Minkowski & newtonian gravity model we are working in an inertial coordinate system (= canonical coordinates) of the underlying Minkowski space. The kinetic energy of each dm probably has to be added to the gravitating mass of each dm.

In this simple model, the gravity potential is indifferent to the acceleration of the elements dm.

                 M

      

             <----- •  dm

             <----- •  m

The test mass m flying along with dm at the surface of M does not "see" the kinetic energy of dm.

An option is to calculate the mass-energy in M in inertial, for the moment, comoving coordinates of m. That might give at least an approximately correct gravity potential.

Where is the event horizon of a rotating mass M located:

1. The event horizon can be defined as the largest radius where the coordinate speed of light, c', is extremely slow in the radial direction toward the center of M.

2. Another definition is where the local time runs extremely slowly relative to the coordinate time.

3. Yet another definition might be that a photon sent from the horizon obtains an extremely large redshift in faraway space.

The definitions 2 and 3 are equivalent. Though, one photon at the sender may be many photons at the receiver.

Conclusions

We outlined what happens in the collapse of a nonrotating star. The end state is a "frozen star", where the coordinate speed of light is extremely slow, and becomes ever slower as time progresses. The end state is not ordered. It is not a "crystal", but an amorphous solid. Like glass at an extremely low temperature.

We also described why the event horizon of a nonrotating frozen star is perfectly spherical – not just in general relativity, but in a wide range of models of gravity.

We sketched how two orbiting frozen stars merge. The event horizon will be rotationally symmetric, for the same reason why the horizon is spherical for a nonrotating frozen star.

We tried to determine the shape of the event horizon of a rotating frozen star, but were not able to find how it should be calculated exactly.

Rogue variation and freely falling observers

A physicist friend of ours suggested that we can make the Einstein-Hilbert action aware of the "correct" kinetic energy by using freely falling observers in measuring the kinetic energy.

https://en.wikipedia.org/wiki/Einstein%E2%80%93Hilbert_action

The lagrangian LM is of the form

       T  -  V

       

       =  kinetic energy  -  potential energy.

Then a particle which we move with a rogue variation (see the blog post May 21, 2024 for the definition of a rogue variation) would gain kinetic energy in the movement, and the Einstein-Hilbert action S would get a larger value when we try to apply a rogue variation. This is a step toward using "canonical coordinates" in general relativity. We fix the spatial coordinates to freely falling observers.

Picking the freely falling observers is problematic

Our best bet is to pick observers which at some "moment" in the entire spacetime are "static" relative to each other. But this is not possible. Close to a fast moving very heavy neutron star, no observer can be static relative to other observers far away.

              •            •

             Q₁          Q₂

What about picking arbitrary freely falling observers? Consider a system where gravity is negligible and in which we have electric charges. A freely falling observer is anyone with a constant speed. Assume that we determine the kinetic energy T of a charge Q from the measurement made by the closest observer. Then T can vary wildly, depending on which observer happens to be close to Q. This is not going to work.

The solution for the electric charge system is to use observers which are static in some coordinate system of Minkowski space. That is equivalent to using canonical coordinates.

If gravity is not negligible, then the orbits of initially static free-falling observers will eventually cross each other. Which observer will we then use to measure the kinetic energy of a nearby particle? The solution is not well defined.

Using the rogue variation to move the freely falling observers, too

There is a much more fundamental problem in using freely falling observers. Let us use a rogue variation to manipulate the metric g around a particle P and a freely falling observer O close to P.

We can manipulate the metric g in such a way that also the freely falling observer O moves along with P. We can keep the metric g essentially unchanged at P and O, and manipulate it at some distance away.

Then O will not notice any change in the kinetic energy of P, even though proper distances of P from other particles change in the rogue variation.

The solution to this problem would be to introduce canonical coordinates, but that is against the fundamental principles of general relativity.

The definition of kinetic energy in general relativity

https://en.wikipedia.org/wiki/Kinetic_energy#General_relativity

Let us have a particle of a mass m whose 4-velocity in the given coordinates is

where τ is the proper time of the particle. Let g be the metric. We define:

Let us have an observer close to the particle, such that the 4-velocity of the observer is uobs. Then the total energy of the particle, as measured by the observer, is

The kinetic energy measured by the observer is defined as:

       Ekin  =  E  -  m c².

Let us check that the formula above agrees with what the observer should measure intuitively.

We assume that the observer sits still at a fixed spatial coordinates. We assume that the coordinate time x⁰, or t, runs at the same speed as the proper time of the observer. Then g₀₀ = -c².

The only non-zero component of uobs is u⁰obs, and its value is 1.

We assume that g has no off-diagonal components. The total energy of the particle, as measured by the observer, is

       E  =  -p₀ u⁰obs 

           =  -m g₀₀ u⁰

           =   m c²  *  dx⁰ / dτ

           =   m c²  *  dt / dτ

           =   m c²  *  1 / sqrt(1  -  v² / c²),

where v is the velocity of the particle as measured by the observer. The total energy agrees with the formula on special relativity. That is, the formula gives the value which the observer intuitively should measure.

Suppose then that the coordinate time t runs C times as fast as the proper time of the observer. Then u⁰obs is C-fold, dx⁰ / dτ is C-fold, and g₀₀ is 1 / C² -fold. The value of E does not change. It is still the same as what the observer intuitively should measure.

The definition of a rogue variation

Let us recapitulate what a rogue variation is. We calculate the Einstein-Hilbert action integral S from a physical system which consists of particles.

A rogue variation aims to change the path of one of the particles, without the Einstein-Hilbert action noticing any change in the kinetic energy of the particle, or in the action integral S.

Let us denote the coordinates by C, and "canonical coordinates", which are determined by proper distances, by Cc.

The metric is represented by a function g on the coordinates C. Let the representation of the metric on the coordinates Cc be gc.

We vary the system by modifying the spatial distances determined by the metric representation g in such a way that, effectively, the spatial coordinate lines of C move relative to the canonical coordinates Cc.

We keep the spacetime geometry of the system constant. That is, the metric representation gc relative to the canonical coordinates Cc stays unchanged. Note that even if the particle's path in the canonical coordinates Cc changes, its personal gravity field stays in the original path! The particle is "decoupled" from its own gravity field.

The path of each particle stays the same in the coordinates C.

                     |                 |

                     |                   \

                     |                   |

                     |                   /    <--- "detour"

                     |                 |

                     • P₁             • P₂

                                           m

         ^  t

         |

          ------> x

Above we have two particles which have a coordinate velocity 0 relative to the coordinates C. The coordinates C were originally identical to the canonical coordinates Cc. Then we modified g in such a way that the particle P₂ did a "detour", if measured in the canonical coordinates.

The metric representation gc does not change. Thus, the action integral of R stays unchanged.

In the matter lagrangian LM, the kinetic energy remains zero, because P₂ stays at the same position in the coordinates C. An observer standing close to P₂ will make a detour along with P₂ and will think that P₂ does not move.

The "potential energy" of P₂ in LM remains as m c², as measured by an observer standing close to P₂.

We conclude that the Einstein-Hilbert action integral S does not change in the rogue variation.

Conclusions

Using freely falling observers to determine the kinetic energy of a particle P does not help. A rogue variation can move also the nearest observers, and the observers think that the kinetic energy of P did not change.

The solution to the problem is to measure the kinetic energy against canonical coordinates, and that is against the principles of general relativity.

Ricci tensor is non-zero in a gravitational wave?

A gravitational wave packet W carries an energy E, but its Ricci tensor R is zero.

The contradiction from the Landau-Lifshitz pseudotensor

https://www.tat.physik.uni-tuebingen.de/~kokkotas/Teaching/GTR_files/GW-Theory-short.pdf

Kostas Kokkotas (2020)  explains how an approximate metric for a gravitational wave packet is constructed. We start from a wave solution

       g  =  η  +  h

of the linearized Einstein wave equations. We assume that g is a perturbation of the flat metric η.

Let us substitute g to the full Einstein field equations. It is not a solution, and in empty space we obtain a residual tensor. The residual tensor is red in the formula:

The red "stress-energy tensor" is the Landau-Lifshitz pseudotensor. It is supposed to describe the energy and momentum density of the wave:

Then we solve another perturbed metric

       g'  =  η  +  h'

from the equation above. The full solution is then

       η  +  h  -  h'.

That is, we form the metric far away by pretending that the wave packet is an electromagnetic wave of the energy E.

Let us have the space filled with initially static infinitesimal test masses m. When the packet W arrives, it pulls on the masses m. The test masses start to get denser around the path of W. That implies that R₀₀ is positive inside the wave W.

We arrived at a contradiction.

Could it be that the test masses in the path of W begin to "overlap", so that the density of any infinitesimal cube of test masses does not grow?

An overlap means that infinitesimal cubes of test masses will go inside each other. An overlap always develops when a long enough time passes. Paths of test masses from different sides of W will eventually cross each other.

It is hard to see how a weak gravitational wave could distort the configuration of the test masses so drastically, so that an "overlap" would develop immediately.

What is the problem in the Landau-Lifshitz trick? The problem is that it is sleight of hand which does not really solve anything. One cannot make the faraway metric to imitate that of an electromagnetic wave of the energy E without introducing a non-zero R₀₀ inside the wave.

The contradiction from Birkhoff's theorem

Let us have a spherically symmetric mass M. We assume that inside M we have devices which send and absorb gravitational waves. The devices cannot be exactly spherically symmetric, but we conjecture that Birkhoff's theorem still approximately holds.

The energy in the gravitational waves inside M cannot alter the metric far away, according to Birkhoff's theorem.

The conclusion is that the faraway metric generated by an almost symmetric configuration of gravitational wave packets W is just like for the analogous electromagnetic waves.

This suggests that an individual wave packet W has a faraway metric like the analogous electromagnetic wave packet. This leads to a contradiction like above.

The contradiction from the ADM formalism

https://en.wikipedia.org/wiki/ADM_formalism

https://physics.stackexchange.com/questions/2597/energy-conservation-in-general-relativity

Certain sources on the Internet claim that the ADM energy, which is defined as a surface integral of the gravity field very far away, is conserved. If the system is spherically symmetric, then this is Birkhoff's theorem.

Let us have a system M which sends a gravitational wave W and absorbs it back. If the claims about the conservation of the ADM energy are correct, then W must have a far-away metric which mimics an equivalent electromagnetic wave. We obtain a contradiction as above.

Exact solutions of gravitational waves

There are known exact solutions for gravitational waves such that R = 0 everywhere. But the waves then are plane waves, and are not physically realistic.

It looks like that realistic gravitational waves always break the Einstein field equations.

In our own Minkowski & newtonian gravity model, gravitational waves do not have a restriction R = 0.

Conclusions

It looks like that the Einstein equations do not have a solution for a gravitational wave packet.

Since we have empirically observed gravitational waves, this is yet another piece of evidence which suggests that the Einstein equations are incorrect.

The metric loses information about magnetism for rotating circle

In our June 4, 2024 blog post we remarked that for a rotating circle, the contribution of the velocity component vy of a mass element dm to gravitomagnetism is asymptotically zero, because of the symmetry. How then can vy significantly affect the magnetic field B of a circular current loop?

We wrote about "private" interactions on September 23, 2023.

The metric g₀₂ is not aware of handedness and loses information

The problem seems to be that the metric of general relativity "loses information", which would be required to calculate the magnetic effect.

                         v

                      <-----  rotation

                   ----------- 

                /                 \

              |         ×           |   rotating circle M or

                \                 /     current loop, charge Q

                  • -----------•

                  |             dm' or dq'

                  v   vy 

 mass element

 dm or

 electron dq

                         ^  V

                         |

                         •  test mass m or

                            test charge q

                      ×   ×  B magnetic field

                      ×   ×

         ^ y

         |

          ------> x

When we calculate the magnetic field B at the test charge q, we use the vector (q, dq) in the calculation. In the diagram, the magnetic field dB caused by vy points into the screen (marked with ×), because dq is approaching, at it is to the left of q.

Also the electron dq' creates a magnetic field dB which points into the screen at q.

In the case of the metric g produced by the rotating circle M, the cross term g₀₂ of

         dt dy

is not aware if the contribution came from the left or from the right. The cross term does not know the "handedness". Since the contributions from each side cancel out each other in g₀₂, they do not produce any gravitomagnetic force on m.

The contribution of vy cancels a large part of the magnetic field B produced by vx. This explains, partially, why gravitomagnetism is stronger by a factor 4 than the analogous magnetism.

Should gravity be aware of handedness?

Is general relativity wrong in ignoring the handedness?

Gauss's law seems to require that for "free" fields, gravitomagnetism must be identical to the analogous magnetism. Can we allow that it differs for a rotating circle?

Could electromagnetism have a 4-fold magnetic field B for a closed current loop?

The magnetic field in electromagnetism is probably dictated by special relativity, and Gauss's law.

For a rotating current loop, there is no outgoing radiation. It might be that the Gauss's law could be satisfied with different strenghts of the magnetic field B. Also, it might be that special relativity would allow the same.

                  ω rotation

                <---

                     ___   Q charge

                  /

                |        × center

                  \____   

                      B 

                  •   •   •

                  |   |   |   E

Suppose that we have a charged half-circle rotating around the center. The magnetic field in this case has to make sure that Gauss's law is satisfied. The lines of of force of (partially induced by dB / dt) the electric field E must not break.

The half-circle gives out electromagnetic radiation.

Let us then combine the half-circle with another half-circle. We have a full circle now, and there no longer is electromagnetic radiation going out.

Could it be that B can change significantly for a full circle?

If that is the case, then nature must somehow know that the circle is now full, and it can strengthen B. We could imagine that if there is no radiation out, then B can somehow grow stronger. But this sounds far-fetched.

Empirically we know that magnetism works the same way for a half-circle and a full circle.

Gravitomagnetism has different fluxes inside the circle and outside it

In electromagnetism, vy reduces the magnetic field outside the current loop, because dB from vy points to the opposite direction to dB from vx.

But inside the loop, these dB point to the same direction.

The metric in gravity loses the information about vy outside the loop. The magnetic field is 4X because of that.

What about inside the loop?

                                                     ^  ω

                                                        \

             |                                  |

          |                   ^ v                |

          |                   |                    |

             |                •                 |

                              m

   ^ y

   |

    ----> x

The information about vy is lost also inside the loop.

In electromagnetism, the effect of vy is to strengthen the magnetic field B inside the loop. This means that the gravitomagnetic field is surprisingly weak inside the loop.

It looks like the hypothetical gravitomagnetic field lines do not close in gravitomagnetism in general relativity!

This may be yet another fatal flaw in general relativity.

If magnetic field lines break, then we have "gravitomagnetic monopoles" in empty space. Does this break the Einstein equations there?

Geodesic deviation equation

                           ^   ^ test masses turn up

                           |   |

                      Bg   

                 ... ----------

                          ×   ×   ×    test masses m

                 ... ----------

                          ×   ×   ×    going into the screen

                 ... ----------

                                ^

                                |

                   gravitomagnetic

                   field lines break here

     ^ z

     |

     •-----> x

     y points into the screen

Suppose that gravitomagnetic field lines break in empty space.

It looks ugly, but we are not able to prove that the Ricci tensor R then necessarily differs from zero. 

If "gravitoelectric" field lines would break, then a cube of initially static test masses will increase in volume, and R₀₀ is then non-zero. But breaking magnetic lines do not seem to cause the same effect.

Conclusions

For a rotating circle, disk, or sphere, the metric of general relativity loses information, compared to the magnetic field B of the analogous electric current loop.

This makes the gravitomagnetic field ugly: gravitomagnetic field lines break. We conclude that general relativity probably has a wrong approach to the problem.

We believe that the correct gravitomagnetic field of a rotating object is fully analogous to the magnetic field of the analogous electric current, but we are not yet able to prove that that is the case.

The metric of a rotating sphere

On May 29, 2024 we used a rogue metric variation to prove that the Einstein field equations do not have any solution for a rotating mass. 

The Einstein field equations have no solution for a rotating mass, but we can still try to build a metric g which is "in the spirit" of general relativity. We can then compare it to the Kerr metric.

Let us recapitulate our argument against the existence.

A rogue metric variation δg proves that the Einstein field equations have no solution for a rotating mass

https://en.wikipedia.org/wiki/Einstein%E2%80%93Hilbert_action

Let g be the solution of the Einstein field equations for a rotating uniform mass sphere M. M is kept from collapsing by pressure and the centrifugal "force".

                       rotation

                          -----> 

                            M

                         •       •

                  •      •       •      •

                  •      •       •       • m ---->  lift higher

                  •      •       •      •

                         •       •           --->  pressure

                                              --->  centrifugal

                                                      "force"

                         <----- 

                      rotation

     ^ y

     |

      ------> x

The action integral S of LM contains:

       ( kinetic energy of m

        - energy of pressure on m

        - m c² )

       * sqrt(-det(g)).

Let us assume that the spatial coordinates are cartesian. Using a rogue metric variation δg, we move the coordinate lines around a particle m a little bit to the right, relative to the spacetime geometry. The integral of R does not change.

The y metric does not change at m. Let the absolute value of the metric of time |g₀₀| ≈ 1 grow by a small amount ε at m, which means that the proper time at m runs by a factor

       1  +  ε / 2

faster.

The coordinate velocity of m does not change. Since proper time now runs faster, an observer standing on m measures that the proper kinetic energy of m decreased by a factor

        1  -  ε.

This is partially compensated by |g₀₀| at m growing by the factor

       1  +  ε / 2.

Thus, the product

       (kinetic energy of m)  *  sqrt(-det(g))

decreases by a factor 1 - ε / 2.

The product

       (energy of pressure on m)  *  sqrt(-det(g))

is the "pressure potential" of m. The product

       m c²  *  sqrt(-det(g))

tells us the newtonian gravity potential of m. If we move m to the right, the newtonian gravity potential increases more than the pressure potential decreases (remember the centrifugal "force" which supports m).

We conclude that the integral of LM decreases if we move m to the right. This contradicts the assumption that g is a solution of the Einstein field equations.

Stretched radial metric grr in cartesian coordinates

Let us have a mass element dm at x, y coordinates (0, 0). We have an observer at coordinates (x, -y), where |y| >> |x|. What is the metric in cartesian coordinates?

We have:

       r²  =  x²  +  y²

  =>

       r dr  =  x dx  +  y dy

  =>

       dr  =  x / r  *  dx  +  y / r  *  dy.

Let dn be the normal to dr:

       dr²  +  dn²  =  dx²  +  dy².

The metric:

       ds²  =  (1  +  hrr) dr²  +  dn²

               =  hrr dr²  +  dr²  +  dn²

               =  hrr dr²  +  dx²  +  dy².

There:

       hrr dr²  =  hrr  * 

                        (x² / r²  *  dx²  

                         + 2 x y / r²  *  dx dy

                         + y² / r²  *  dy²).

Lorentz transforming a Schwarzschild metric perturbation

https://en.wikipedia.org/wiki/Lorentz_transformation

We want to calculate the metric produced by a moving mass element m. The metric perturbation h created by m is approximately Schwarzschild, and in many cases we can obtain an approximate solution of the Einstein equations by summing metric perturbations.

                       ^  y

                       |

                       |

                 m  ●----> vx  

                       |\     

                       |  \ 

                       |    \

                       vy    v   v

                            R

                                • observer  (x, -y)

The mass element m is at x, y coordinates (0, 0). The observer is at (x, -y), where

       |x|  <<  |y|.

We denote x² + y² = R².

The components of the velocity v are vx and vy.

Let η be the flat metric. We want to transform, at the observer,

       η  +  h

to coordinates moving at a velocity v. We will transform twice, for each component of v. Let us calculate the transformation for vx.

https://en.wikipedia.org/wiki/Schwarzschild_metric

The metric at the observer is:

       c² dτ²  =  -(1  -  rs / r) c² dt²

                        + (1  +  rs / r  *  x² / r²)  *  dx²

                        + (1  +  rs / r  *  y² / r²)  *  dy²

                        + 2 rs / r  *  x y / r²  *  dx dy

                        + dz²,

where rs = 2 G m / c² and r = -y. Lorentz says:

       dt   =  dt'  +  vx / c²  *  dx',

       dx  =  dx'  +  vx dt',

       dy  =  dy',

       dz  =  dz',

       c² dτ²  ≈  -(1  -  vx² / c²) (1  -  rs / r) c² dt'²

                        + (1  +  rs / r  *  x'² / r²)  *  dx'²

                        + (1  +  rs / r  *  y'² / r²)  *  dy'²

                        + 2 rs / r  *  x' y' / r²  *  dx' dy'

 

                        + dz'²

    

                        + (2 vx  -  2 vx  +  rs / r * 2 vx) dt' dx',

where we used the facts that x' ≈ x, y' = y, and that the product rs vx * |x|/ r is very small. The value of r is approximately the same in the new coordinates.

The difference to the static metric is

       vx² dt'²

and the cross term

       2 vx rs / r  *  dt' dx'.

The velocity vx makes the metric perturbation of time t to "spill over" to the cross term dt' dx'.

The velocity vy, obviously, has a similar effect. A difference is that also the perturbation of the y metric spills over to the cross term.

Summing the effects of vx for a rotating circle

We must sum the term for each mass element dm:

       rs / r  *  vx  *  dt' dx'.

Let

       r'  =  distance (observer, center of circle).

            •  dm'

               \

                  \

                     \

                       ×

                       |  \                    ^   ω

                       |     \   R           /

                       |        \

                       |          • dm       M

                           α

                       r' = distance(×, observer)

          

                       •  observer

Let us integrate it for a circle of a radius R, which turns at an angular velocity ω. The mass of the circle is M. The distance of dm from the observer is:

       r  =  sqrt( (r'  -  R cos(α))² + R² sin²(α) )

           ≈  r' (1  -  R / r'  *  cos(α)).

Since vx has opposite values for dm and its mirror image dm' relative to the center of the circle, we can sum their contribution. We have:

       rs   =  2 G / c²  *  M / (2 π)  *  dα,

       vx  =  R ω cos(α),

                    π / 2

       h₀₁  =     ∫  

                  -π / 2

                     2 G / c²  *  M / (2 π) 

                     * R ω cos(α)

                     * 1 / r'  *  (1  +  R / r'  * cos(α)

                                       - 1  +  R / r'  *  cos(α))

                     * dα.

The integral

           π / 2

            ∫  2 cos²(α) dα  =  π.

       -π / 2

We get:

       h₀₁  =  G / c²  *  M ω R² / r'²

              =  G / c²  *  J  / r'²,

where J is the angular momentum of the circle.

The part of the metric perturbation due to the rotation of the disk or the sphere is:

      0                        G / c² * J / r'²    0      0

      G / c² * J / r'²    0                        0      0

      0                        0                        0      0

      0                        0                        0      0.

Summing the effects of vy for a rotating circle

We studied this extensively in August 2023.

The symmetry of the disk makes the cross term of t' y' in the metric zero if the observer is at (0, -y) in the diagram.

                       ^ y'

                       |

                      ● -----> x'

                     M  (0, 0)

                      

                      r'

              

                      • observer (0, -y')

                 <----> cross term of t' x'

When the observer moves to the x direction, the cross term will have a non-zero value. But is the value negligible?

Let the observer move to a position (x₀', -y₀'), where |x'| is small. The "radial" coordinates there are rotated through an angle x₀' / y₀' = β.

       x''  =  x'  +  β y',

       y''  =  y'   -  β x'.

Let us have a cross term in the metric at the new position:

       C t' x''  =  C t' x'  + C t' β y'.

The cross term t' y' in the old coordinates is

       C x₀' / y₀'  *  t' y'.

We have

       C  =  2 rs / r  *  vx.

If we make y₀' large, then the cross term of t' and y' is negligible relative to the cross term of t' and x'.

It looks like that vy would not contribute at all. The reason is that each dm has a centripetal acceleration.

We are not sure if this is the correct "approximate" solution in general relativity. If the mass M is lightweight, then a negative pressure keeps the parts dm in the circular orbit. If we let a rogue variation to move a part dm farther from the center of the circle, then the action integral of the matter lagrangian LM decreases. That shows that the Einstein field equations have no solution.

The fact that the t' y' cross term is negligible is due to the same reason why the Einstein equations have no solution! It does not sound like a proper approximate solution!

Test mass m acceleration due to the rotation of M

Now we can calculate the gravitomagnetic effects on m.

                     J

           |       ● M

           v ω

                     r'

                     ^  V

                     |

                     • m

     ^ y

     |

      -------> x

https://en.wikipedia.org/wiki/Geodesics_in_general_relativity

The x coordinate acceleration is

       d²x / dτ²  =  -Γ¹₀₀  *  dt / dτ  *  dt / dτ

                            - 2 Γ¹₀₂  *  dt / dτ  *  dy / dτ

                            - Γ¹₂₂  *  dy / dτ  *  dy / dτ

                        =  -2 * 1/2 g¹¹ dg₀₁ / dy  *  1  *  V

                            - 2 * 1/2 g⁰¹ dg₀₀ / dy  *  1  *  V

                        ≈  -V dg₀₁ / dy

                        =  V  *  G / c²  *  2 J  / r'³.

The gravitomagnetic moment, as defined by our August 10, 2023 blog posts, is 2 J. It is 4 times the analogous magnetic moment of a rotating electric charge. This is probably the origin of the strange 4-fold gravitomagnetism in literature.

Tangential movement of a test mass

Above we calculated the effect of vx if the test mass m approaches M. What about m moving tangentially?

                            ●  M

     

                             • -----> V

                            m

       d²y / dτ²  =  -Γ²₀₀  *  dt / dτ  *  dt / dτ

                            - 2 Γ²₀₁  *  dt / dτ  *  dx / dτ,

       Γ²₀₀  =  1/2 g²²  *  -dg₀₀ / dy

                =  1/2  *  (1  +  rs / r)  *  rs / r²

                =  1/2 rs / r²  +  1/2 rs² / r³,

       Γ²₀₁  =  1/2 g²²  *  -dg₀₁ / dy

                ≈  1/2  *  -G / c²  *  2 J  / r'³.

                =  -G / c²  *  J / r'³.

Since both sides in the equation for d²y / dτ² contain ... / dτ², we can instead write .../ dt².

The y coordinate acceleration due to vx and V is

       d²y / dt²  =  V  *  G / c²  *  2 J  / r'³.

This agrees with the previous section. The gravitomagnetic moment is 2 J.

On top of that we have the regular

       1/2 rs / r²  =  G M / c²  *  c²  *  1 / r²

                         =  G M / r²

gravity acceleration.

But is the y coordinate "natural" here? If we make coordinate lines to "bulge", we can obtain spurious coordinate accelerations, which depend on V.

A single moving mass element dm creates a time-dependent metric. We have forgotten that in the analysis above!

The gravitomagnetic field of a single moving mass

Let us calculate a very simple example.

              M ● ----> v

                   r

              m • -----> v

       ^ y

       |

        • ----> x

 observer

The mass M is moving at a velocity v to the x direction, and so is the test mass m.

If both were static in our coordinates, then a static observer would measure a y acceleration of

       d²y / dt²  =  G M / r²,

where r is the Schwarzschild radial coordinate of m.

But proper time in the system M, m is slower than for the observer. He measures an acceleration of

       d²y / dt²  =  (1  -  v² / c²) G M / r².

The term

       v  *  v / c²  *  G M / r²

can be interpreted as a "magnetic" effect. It corresponds to a gravitomagnetic field of

       v / c²  *  G M / r²,

which is analogous to a magnetic field

       B  =  v / c²  *  E.

There is no factor 4. 

Let us use the geodesic equation to calculate the acceleration. The metric in the laboratory coordinates is:

       c² dτ²  ≈  -(1  -  v² / c²) (1  -  rs / r) c² dt²

                        + (1  -  v² / c²)  *  dx²

                        + (1  +  rs / r)  *  dy²

 

                        + dz²

    

                        + rs / r  *  2 v dt dx,

where

       rs  =  2 G M / c².

The acceleration:

       d²y / dt²  =  -Γ²₀₀  *  dt / dt  *  dt / dt

                            -2 Γ²₀₁  *  dt / dt  *  dx / dt

                        =  (1  +  v² / c²)  G M / r²

                             - 2  *  1/2 g²²  *  -dg₀₁ / dy  *  v

                        ≈  (1  +  v² / c²) G M / r²

                            - 1  *  1  *  rs / r²  *  v  * v

                        =  G M / r²

                            - v² G M / c².

                  M

                   ● --> v

                   ^  V

                   |

                   •  m

   ^ y

   |

    -----> x

Let us calculate the x acceleration.

       d²x / dτ²  =  -2 Γ¹₀₂  *  dt / dτ  *  dy / dτ,

                        =  -2 * 1/2 g¹¹ dg₀₁ / dy  *  1  *  V

                            - 2 * 1/2 g⁰¹ dg₀₀ / dy  *  1  *  V

                        =  -V d(v rs / r) / dy

                        =  V v  *  2 G M / c²  * 1 / r².

What is wrong in the above calculation?

An obvious problem is that dm is accelerating. Can we sum the metric perturbations as if the mass elements dm were moving at a constant velocity?

We wrote at length about this problem in August 2023.

Now we know that the Einstein equations do not have a solution at all for a rotating system, because it is "dynamic".

Conclusions

If we want to preserve Gauss's law for newtonian gravity for a gravitational wave, we need magnetic gravity which is exactly analogous to magnetism. The condition R₀₀ = 0 states Gauss's law for gravity.

In the above calculations, we were able to track the origin of the strange factor 4 in literature, of the gravitomagnetic effect of a rotating sphere.

If gravitomagnetism for gravitational waves is exactly analogous to electromagnetism, then it would be surprising if "static" gravitomagnetic fields differ from electromagnetism by the factor 4. How does nature know to make the effect 4-fold in certain configurations?

We will investigate this in the next blog post.